Barth surface
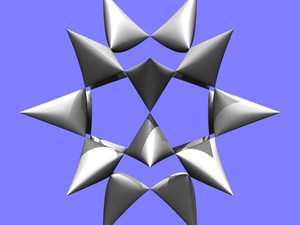
Real points of the Barth Sextic.

Barth Decic
In algebraic geometry, a Barth surface is one of the complex nodal surfaces in 3 dimensions with large numbers of double points found by Wolf Barth (1996). Two examples are the Barth sextic of degree 6 with 65 double points, and the Barth decic of degree 10 with 345 double points.
Some admit icosahedral symmetry.
For degree 6 surfaces in P3, Jaffe & Ruberman (1997) showed that 65 is the maximum number of double points possible. The Barth sextic is a counterexample to an incorrect claim by Francesco Severi in 1946 that 52 is the maximum number of double points possible.
See also
References
- Barth, W. (1996), "Two projective surfaces with many nodes, admitting the symmetries of the icosahedron", Journal of Algebraic Geometry, 5 (1): 173–186, MR 1358040.
- Jaffe, David B.; Ruberman, Daniel (1997), "A sextic surface cannot have 66 nodes", Journal of Algebraic Geometry, 6 (1): 151–168, MR 1486992.
External links
This article is issued from Wikipedia - version of the 10/27/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.