Birectified 16-cell honeycomb

Birectified 16-cell honeycomb
(No image)
Type	Uniform honeycomb
Schläfli symbol	t2{3,3,4,3}
Coxeter-Dynkin diagram	=
4-face type	Rectified tesseract Rectified 24-cell
Cell type	Cube Cuboctahedron Tetrahedron
Face type	{3}, {4}
Vertex figure	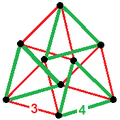{3}×{3} duoprism
Coxeter group	${\tilde {F}}_{4}$ = [3,3,4,3] ${\tilde {B}}_{4}$ = [4,3,3^1,1] ${\tilde {D}}_{4}$ = [3^1,1,1,1]
Dual	?
Properties	vertex-transitive

In four-dimensional Euclidean geometry, the birectified 16-cell honeycomb (or runcic tesseractic honeycomb) is a uniform space-filling tessellation (or honeycomb) in Euclidean 4-space.

Symmetry constructions

There are 3 different symmetry constructions, all with 3-3 duoprism vertex figures. The ${\tilde {B}}_{4}$ symmetry doubles on ${\tilde {D}}_{4}$ in three possible ways, while ${\tilde {F}}_{4}$ contains the highest symmetry.

Affine Coxeter group	${\tilde {F}}_{4}$ [3,3,4,3]	${\tilde {B}}_{4}$ [4,3,3^1,1]	${\tilde {D}}_{4}$ [3^1,1,1,1]
Coxeter diagram
Vertex figure
Vertex figure symmetry	[3,2,3] (order 36)	[3,2] (order 12)	[3] (order 6)
4-faces
Cells

Related honeycombs

The [3,4,3,3], , Coxeter group generates 31 permutations of uniform tessellations, 28 are unique in this family and ten are shared in the [4,3,3,4] and [4,3,3^1,1] families. The alternation (13) is also repeated in other families.

F4 honeycombs  Extended symmetry Extended diagram Order Honeycombs [3,3,4,3] ×1 1, 3, 5, 6, 8, 9, 10, 11, 12 [3,4,3,3] ×1 2, 4, 7, 13, 14, 15, 16, 17, 18, 19, 20, 21, 22 23, 24, 25, 26, 27, 28, 29 [(3,3)[3,3,4,3^*]] =[(3,3)[3^1,1,1,1]]=[3,4,3,3] = = ×4 (2), (4), (7), (13)

The [4,3,3^1,1], , Coxeter group generates 31 permutations of uniform tessellations, 23 with distinct symmetry and 4 with distinct geometry. There are two alternated forms: the alternations (19) and (24) have the same geometry as the 16-cell honeycomb and snub 24-cell honeycomb respectively.

B4 honeycombs
Extended symmetry	Extended diagram	Order	Honeycombs
[4,3,3^1,1]:		×1	5, 6, 7, 8
<[4,3,3^1,1]>: ↔[4,3,3,4]	↔	×2	9, 10, 11, 12, 13, 14, (10), 15, 16, (13), 17, 18, 19
[3[1^+,4,3,3^1,1]] ↔ [3[3,3^1,1,1]] ↔ [3,3,4,3]	↔ ↔	×3	1, 2, 3, 4
[(3,3)[1^+,4,3,3^1,1]] ↔ [(3,3)[3^1,1,1,1]] ↔ [3,4,3,3]	↔ ↔	×12	20, 21, 22, 23

There are ten uniform honeycombs constructed by the ${\tilde {D}}_{4}$ Coxeter group, all repeated in other families by extended symmetry, seen in the graph symmetry of rings in the Coxeter–Dynkin diagrams. The 10th is constructed as an alternation. As subgroups in Coxeter notation: [3,4,(3,3)^*] (index 24), [3,3,4,3^*] (index 6), [1⁺,4,3,3,4,1⁺] (index 4), [3^1,1,3,4,1⁺] (index 2) are all isomorphic to [3^1,1,1,1].

The ten permutations are listed with its highest extended symmetry relation:

D4 honeycombs
Extended symmetry	Extended diagram	Extended group	Honeycombs
[3^1,1,1,1]		${\tilde {D}}_{4}$	(none)
<[3^1,1,1,1]> ↔ [3^1,1,3,4]	↔	${\tilde {D}}_{4}$×2 = ${\tilde {B}}_{4}$	(none)
<2[^1,13^1,1]> ↔ [4,3,3,4]	↔	${\tilde {D}}_{4}$×4 = ${{\tilde {C}}}_{4}$	1, 2
[3[3,3^1,1,1]] ↔ [3,3,4,3]	↔	${\tilde {D}}_{4}$×6 = ${\tilde {F}}_{4}$	3, 4, 5, 6
[4[^1,13^1,1]] ↔ [[4,3,3,4]]	↔	${\tilde {D}}_{4}$×8 = ${{\tilde {C}}}_{4}$×2	7, 8, 9
[(3,3)[3^1,1,1,1]] ↔ [3,4,3,3]	↔	${\tilde {D}}_{4}$×24 = ${\tilde {F}}_{4}$
[(3,3)[3^1,1,1,1]]^+ ↔ [3^+,4,3,3]	↔	½${\tilde {D}}_{4}$×24 = ½${\tilde {F}}_{4}$	10

See also

Regular and uniform honeycombs in 4-space:

• Tesseractic honeycomb
• 16-cell honeycomb
• 24-cell honeycomb
• Rectified 24-cell honeycomb
• Truncated 24-cell honeycomb
• Snub 24-cell honeycomb
• 5-cell honeycomb
• Truncated 5-cell honeycomb
• Omnitruncated 5-cell honeycomb

Notes

References

• Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6
  • (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III, [Math. Zeit. 200 (1988) 3-45]
• George Olshevsky, Uniform Panoploid Tetracombs, Manuscript (2006) (Complete list of 11 convex uniform tilings, 28 convex uniform honeycombs, and 143 convex uniform tetracombs)
• Klitzing, Richard. "4D Euclidean tesselations". x3o3x *b3x *b3o, x3o3o *b3x4o, o3o3x4o3o - bricot - O106

Fundamental convex regular and uniform honeycombs in dimensions 3–10 (or 2-9)
Family	${\tilde {A}}_{n-1}$	${\tilde {C}}_{n-1}$	${\tilde {B}}_{n-1}$	${\tilde {D}}_{n-1}$	${\tilde {G}}_{2}$ / ${\tilde {F}}_{4}$ / ${\tilde {E}}_{n-1}$
Uniform tiling	{3^[3]}	δ3	hδ3	qδ3	Hexagonal
Uniform convex honeycomb	{3^[4]}	δ4	hδ4	qδ4
Uniform 5-honeycomb	{3^[5]}	δ5	hδ5	qδ5	24-cell honeycomb
Uniform 6-honeycomb	{3^[6]}	δ6	hδ6	qδ6
Uniform 7-honeycomb	{3^[7]}	δ7	hδ7	qδ7	222
Uniform 8-honeycomb	{3^[8]}	δ8	hδ8	qδ8	133 • 331
Uniform 9-honeycomb	{3^[9]}	δ9	hδ9	qδ9	152 • 251 • 521
Uniform 10-honeycomb	{3^[10]}	δ10	hδ10	qδ10
Uniform n-honeycomb	{3^[n]}	δn	hδn	qδn	1k2 • 2k1 • k21