Doo–Sabin subdivision surface
In computer graphics, Doo–Sabin subdivision surface is a type of subdivision surface based on a generalization of bi-quadratic uniform B-splines. It was developed in 1978 by Daniel Doo and Malcolm Sabin.[1][2]
This process generates one new face at each original vertex, n new faces along each original edge, and n x n new faces at each original face. A primary characteristic of the Doo–Sabin subdivision method is the creation of four faces around every vertex. A drawback is that the faces created at the vertices are not necessarily coplanar.
Evaluation
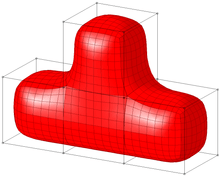
Doo–Sabin surfaces are defined recursively. Each refinement iteration replaces the current mesh with a smoother, more refined mesh, following the procedure described in.[2] After many iterations, the surface will gradually converge onto a smooth limit surface. The figure below show the effect of two refinement iterations on a T-shaped quadrilateral mesh.

Just as for Catmull–Clark surfaces, Doo–Sabin limit surfaces can also be evaluated directly without any recursive refinement, by means of the technique of Jos Stam.[3] The solution is, however, not as computationally efficient as for Catmull-Clark surfaces because the Doo–Sabin subdivision matrices are not in general diagonalizable.
See also
- Expansion - Equivalent geometric operation - truncates vertices and beveling edges.
- Conway polyhedron notation - A set of related topological polyhedron and polygonal mesh operators.
External links
- ↑ D. Doo: A subdivision algorithm for smoothing down irregularly shaped polyhedrons, Proceedings on Interactive Techniques in Computer Aided Design, pp. 157 - 165, 1978 (pdf)
- ↑ 2.0 2.1 D. Doo and M. Sabin: Behavior of recursive division surfaces near extraordinary points, Computer-Aided Design, 10 (6) 356–360 (1978), (doi, pdf)
- ↑ Jos Stam, Exact Evaluation of Catmull–Clark Subdivision Surfaces at Arbitrary Parameter Values, Proceedings of SIGGRAPH'98. In Computer Graphics Proceedings, ACM SIGGRAPH, 1998, 395–404 (pdf, downloadable eigenstructures)