End extension
In model theory and set theory, which are disciplines within mathematics, a model 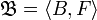 of some axiom system of set theory
of some axiom system of set theory 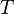 in the language of set theory is an end extension of
in the language of set theory is an end extension of 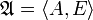 , in symbols
, in symbols 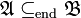 , if
, if
-
 is a substructure of
is a substructure of 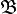 , and
, and -
 whenever
whenever 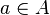 and
and 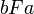 hold, i.e., no new elements are added by
hold, i.e., no new elements are added by 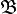 to the elements of
to the elements of  .
.
The following is an equivalent definition of end extension:  is a substructure of
is a substructure of 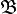 , and
, and 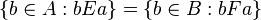 for all
for all 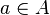 .
.
For example,  is an end extension of
is an end extension of 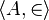 if
if 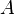 and
and 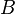 are transitive sets, and
are transitive sets, and 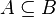 .
.
This article is issued from Wikipedia - version of the 1/28/2009. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.