Saddle point

A saddle point on the graph of z=x²−y² (in red)

Saddle point between two hills (the intersection of the figure-eight $z$-contour)

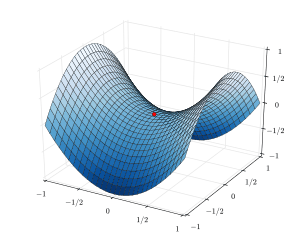
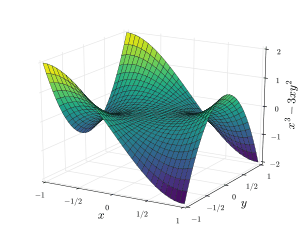
In mathematics, a saddle point is a point in the domain of a function where the slopes (derivatives) of orthogonal function components defining the surface become zero (a stationary point) but are not a local extremum on both axes. The saddle point will always occur at a relative minimum along one axial direction (between peaks) and where the crossing axis is a relative maximum.

The name derives from the fact that the prototypical example in two dimensions is a surface that curves up in one direction, and curves down in a different direction, resembling a riding saddle or a mountain pass between two peaks forming a landform saddle. In terms of contour lines, a saddle point in two dimensions gives rise to a contour graph or trace that appears to intersect itself—such conceptually might form a 'figure eight' around both peaks; assuming the contour graph is at the very 'specific altitude' of the saddle point in three dimensions.

Mathematical discussion

A simple criterion for checking if a given stationary point of a real-valued function F(x,y) of two real variables is a saddle point is to compute the function's Hessian matrix at that point: if the Hessian is indefinite, then that point is a saddle point. For example, the Hessian matrix of the function $z=x^2-y^2$ at the stationary point $(0,0)$ is the matrix

$\begin{bmatrix} 2 & 0\\ 0 & -2 \\ \end{bmatrix}$

which is indefinite. Therefore, this point is a saddle point. This criterion gives only a sufficient condition. For example, the point $(0,0)$ is a saddle point for the function $z=x^4-y^4,$ but the Hessian matrix of this function at the origin is the null matrix, which is not indefinite.

In the most general terms, a saddle point for a smooth function (whose graph is a curve, surface or hypersurface) is a stationary point such that the curve/surface/etc. in the neighborhood of that point is not entirely on any side of the tangent space at that point.

The plot of y = x³ with a saddle point at 0

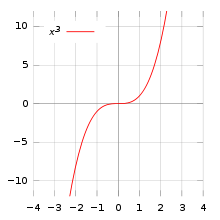
In one dimension, a saddle point is a point which is both a stationary point and a point of inflection. Since it is a point of inflection, it is not a local extremum.

Saddle surface

Hyperbolic paraboloid

A model of an elliptic hyperboloid of one sheet

A monkey saddle

A saddle surface is a smooth surface containing one or more saddle points.

Classical examples of two-dimensional saddle surfaces in the Euclidean space are second order surfaces, the hyperbolic paraboloid $z=x^2-y^2$ (which is often referred to as the saddle surface or "the standard saddle surface") and the hyperboloid of one sheet. The Pringles potato chip or crisp is an everyday example of a hyperbolic paraboloid shape.

Saddle surfaces have negative Gaussian curvature which distinguish them from convex/elliptical surfaces which have positive Gaussian curvature. A classical third-order saddle surface is the monkey saddle.

The PhD thesis of Grigori Perelman, titled "Saddle surfaces in Euclidean spaces," was devoted to saddle surfaces.^[1]

Other uses

In dynamical systems, if the dynamic is given by a differentiable map f then a point is hyperbolic if and only if the differential of ƒ ⁿ (where n is the period of the point) has no eigenvalue on the (complex) unit circle when computed at the point. Then a saddle point is a hyperbolic periodic point whose stable and unstable manifolds have a dimension that is not zero.

In a two-player zero sum game defined on a continuous space, the equilibrium point is a saddle point.

A saddle point of a matrix is an element which is both the largest element in its column and the smallest element in its row.

For a second-order linear autonomous system, a critical point is a saddle point if the characteristic equation has one positive and one negative real eigenvalue.^[2]

See also

• Saddle-point method is an extension of Laplace's method for approximating integrals
• Extremum
• Derivative test
• Hyperbolic equilibrium point
• Minimax theorem
• Max–min inequality

Notes

1. Перельман, Григорий Яковлевич (1990). Седловые поверхности в евклидовых пространствах: Автореф. дис. на соиск. учен. степ. канд. физ.-мат. наук (in Russian). Ленинградский Государственный Университет. [Perelman, Gregory (1990). Saddle surfaces in Euclidean spaces: Author. Dis. on soisk. scientists. step. cand. Sci. Science (in Russian). Leningrad State University.]
2. von Petersdorff 2006

References

• Gray, Lawrence F.; Flanigan, Francis J.; Kazdan, Jerry L.; Frank, David H; Fristedt, Bert (1990), Calculus two: linear and nonlinear functions, Berlin: Springer-Verlag, p. 375, ISBN 0-387-97388-5
• Hilbert, David; Cohn-Vossen, Stephan (1952), Geometry and the Imagination (2nd ed.), New York: Chelsea, ISBN 978-0-8284-1087-8
• von Petersdorff, Tobias (2006), "Critical Points of Autonomous Systems", Differential Equations for Scientists and Engineers (Math 246 lecture notes)
• Widder, D. V. (1989), Advanced calculus, New York: Dover Publications, p. 128, ISBN 0-486-66103-2
• Agarwal, A., Study on the Nash Equilibrium (Lecture Notes)

Further reading

• Hilbert, David; Cohn-Vossen, Stephan (1952). Geometry and the Imagination (2nd ed.). Chelsea. ISBN 0-8284-1087-9.