Great dodecahemidodecahedron
| Great dodecahemidodecahedron | |
|---|---|
 | |
| Type | Uniform star polyhedron |
| Elements | F = 18, E = 60 V = 30 (χ = −12) |
| Faces by sides | 12{5/2}+6{10/3} |
| Wythoff symbol | 5/3 5/2 | 5/3 (double covering) |
| Symmetry group | Ih, [5,3], *532 |
| Index references | U70, C86, W107 |
| Dual polyhedron | Great dodecahemidodecacron |
| Vertex figure |  5/2.10/3.5/3.10/3 |
| Bowers acronym | Gidhid |
In geometry, the great dodecahemidodecahedron is a nonconvex uniform polyhedron, indexed as U70. Its vertex figure is a crossed quadrilateral.
It is the only nonconvex uniform polyhedron whose faces are all non-convex regular polygons (star polygons), namely the star polygons {5/2} and {10/3}, besides the regular small stellated dodecahedron {5/2,5} and great stellated dodecahedron {5/2,3}.
It is a hemipolyhedron with 6 decagrammic faces passing through the model center.
Related polyhedra
Its convex hull is the icosidodecahedron. It also shares its edge arrangement with the great icosidodecahedron (having the pentagrammic faces in common), and with the great icosihemidodecahedron (having the decagrammic faces in common).
 Great icosidodecahedron |
 Great dodecahemidodecahedron |
 Great icosihemidodecahedron |
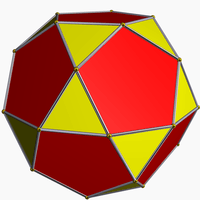 Icosidodecahedron (convex hull) |
Filling
There is some controversy on how to colour the faces of this polyhedron. Although the common way to fill in a polygon is to just colour its whole interior, this can result in some filled regions hanging as membranes over empty space. Hence, the "neo filling" is sometimes used instead as a more accurate filling. In the neo filling, orientable polyhedra are filled traditionally, but non-orientable polyhedra have their faces filled with the modulo-2 method (only odd-density regions are filled in).[1]
 Traditional filling |
 "Neo filling" |
